Okay. So the first order of business I think is the find what "x" is. There are two points that have a sine of -3/5 (one in quadrant 3 and one in quadrant 4). So let's keep that in mind when solving.
sin(x) = -3/5
We can just take the inverse sine of -3/5 to find "x".
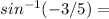 -36.87° approx.
-36.87° approx.
Now keep in mind that -36.87° is in quadrant 4. So its partner in quadrant 3 would be...
180+36.87=216.87° approx.
Last step...
tan(216.87/2)= -3.00 approx.
answer: -3.00 approx.