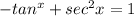Question

Prove the identity step by step

-tan^{2} x + sec^{2} x = 1

Answer 1

`-tan^x + sec^2x = 1` is proved

Solution:

We have to prove that,

`-tan^x + sec^2x = 1`

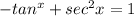
By the trignometric identity,

`sin^2x + cos^2 x = 1`

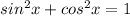
Divide both the sides by
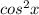`cos^2x` in above identity,

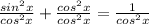`(sin^2x)/(cos^2x) + (cos^2x)/(cos^2x) = (1)/(cos^2x)` --- eqn 1

We know that by definition of tan,

`tan x = (sinx)/(cosx)`

Therefore,

`tan^2x = (sin^2x)/(cos^2x)`

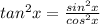
Apply the above in eqn 1

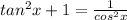`tan^2x + 1 = (1)/(cos^2x)` ---- eqn 2

By definition of cosine,

`cosx = (1)/(secx)`

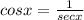
Therefore,

`cos^2x = (1)/(sec^2x)`

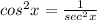
Apply the above in eqn 2

`tan^2x + 1 = sec^2x`

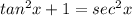
On rewriting we get,

`sec^2x - tan^2x = 1\\`

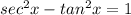
Thus the given identity is proved step by step