Question

Please help!!!!

I will be truly gratefully for whoever helps me
(You don't have to do the whole 2 parts but I hope you might, but atleast try to do the first part)

Answer 1

A)

the sum of all interior angles on any polygon is 180( n - 2 ), where is n = sides in the polygon.

now, this is a quadrilateral, so it has 4 sides, so the sum of all interior angles in degrees for it will be 180( 4 - 2), namely 360°, we know the angle at B is 90°, thus

`\bf \stackrel{B}{90}+\stackrel{A}{2x}+\stackrel{D}{3x}+\stackrel{C}{x}~~=~~360\implies 90+6x=360\implies 6x=270 \\\\\\ x = \cfrac{270}{6}\implies x = 45`

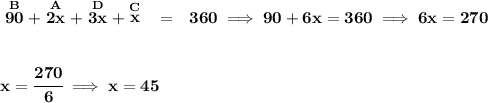
B)

well, if we dilate or blow up the quadrilateral, the angles always remain the same, same if we shrink it, now, points A and B are colinear, they're lying on the same line, we know B is 90°, hmmm what is A anyway? well, A is 2x, but we know x = 45, thus A is 2(45) = 90°.

since the angle at A is 90° and the angle at B is 90°, and both stem from the same colinear line, the lines AD and BC are perpendicular to AB and also parallel lines, no matter how you scale them, down or up.

Answer 2

Explanation:

e sjakbwhshahjsjJakqkak