Question

A space-ship negotiates a circular turn of radius 2200 km at a speed of 27800 km/h.

A) What is the magnitude of the angular velocity?
B) What is the magnitude of the radial acceleration?
C) What is the magnitude of the tangential acceleration?

Answer 1

given,

Radius of circular turn (r)= 2200 km = 2200 x 10³ m

speed of the spaceship = 27800 km/h

v = 27800 x 0.278

v = 7728.4 m/s

a) magnitude of angular velocity

we know,

v = r ω

`\omega = (v)/(r)`

`\omega = (7728.4)/(2200 * 10^3)`

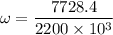
ω = 3.51 x 10⁻³ rad/s

b) magnitude of radial acceleration

`a_r = (v^2)/(r)`

`a_r = (7728.4^2)/(2200 * 10^3)`

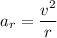
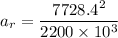
a_r = 27.149 rad/s²

c) spaceship is moving with constant velocity, the tangential acceleration of the spaceship is equal to zero.

a_t = 0 m/s²