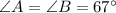Question

The isosceles trapezoid is part of an

isosceles triangle with a 46° vertex angle.
Enter the measure of an acute base angle of
the trapezoid.

Answer 1

Therefore, the measure of an acute base angle of the trapezoid are

`\angle A=\angle B=67\°`

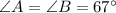
Explanation:

Given:

Consider a Triangle ΔABC,with vertex angle as ∠A,

∠ A = 46°

DECB is an Isosceles trapezoid is part of an Isosceles triangle,

To Find:

∠ B = ∠C = ?

Solution:

As the Triangle,ΔABC is an Isosceles Triangle

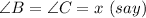`\angle B=\angle C=x\ (say)`........Base angles are equal

Also we have,

Triangle sum property:

In a Triangle sum of the measures of all the angles of a triangle is 180°.

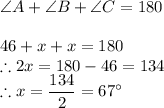
`\angle A+\angle B+\angle C=180\\\\46+x+x=180\\\therefore 2x =180-46=134\\\therefore x=(134)/(2)=67\°`

Therefore, the measure of an acute base angle of the trapezoid are

`\angle A=\angle B=67\°`