Answer:

Explanation:
First, find the rate of change [slope]:
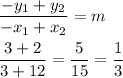
Then plug these coordinates into the Slope-Intercept Formula instead of the Point-Slope Formula since you get it swiftly that way. It does not matter which ordered pair you choose:
2 = ⅓[12] + b
4
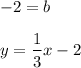
If you want it in Standard Form:
y = ⅓x - 2
- ⅓x - ⅓x
_________
−⅓x + y = −2 [We do not want fractions in our standard equation, so multiply by the denominator to get rid of it.]
−3[−⅓x + y = −2]
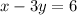
_______________________________________________
−3 = ⅓[−3] + b
−1
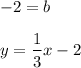
If you want it in Standard Form:
y = ⅓x - 2
- ⅓x - ⅓x
_________
−⅓x + y = −2 [We do not want fractions in our standard equation, so multiply by the denominator to get rid of it.]
−3[−⅓x + y = −2]
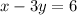
** You see? I told you it did not matter which ordered pair you choose because you will always get the exact same result.
I am joyous to assist you anytime.