Question

A construction company needs to remove tons of dirt from a construction site. They can remove tons of dirt each hour. How long will take to remove the dirt.

Answer 1

Question:

A construction company needs to remove 24 tons of dirt from a construction site. They can remove 3/4 tons of dirt each hour. How long will it take to remove dirt

Answer:

It takes 32 hours to remove the dirt

Explanation:

Given:

Total amount dirt to be removed = 24 tons

Dirt that can removed in one hour = 3/4 tons

To Find:

Time taken to remove all the dirt =?

Solution:

Let the time taken to remove the dirt from the company be x.

Then

x =
`\frac{ \text { total amount of dirt in the company}}{\text{ amount of dirt removed in one hour}}`

Substituting the given values , we get

x =
`(24)/((3)/(4))`

x =
`24* (4)/(3)`

x =
`(96)/(3)`

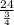
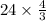
x= 32