Question

Prove that if $w,z$ are complex numbers such that $|w|=|z|=1$ and $wz\\e -1$, then $\frac{w+z}{1+wz}$ is a real number.

Answer 1

See proof below

Explanation:

Let
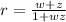`r=(w+z)/(1+wz)`. If w=-z, then r=0 and r is real. Suppose that w≠-z, that is, r≠0.

Remember this useful identity: if x is a complex number then
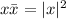`x\bar{x}=|x|^2` where
`\bar{x}` is the conjugate of x.

Now, using the properties of the conjugate (the conjugate of the sum(product) of two numbers is the sum(product) of the conjugates):

`\frac{r}{\bar{r}}=(w+z)/(1+wz) \left(\frac{1+\bar{w}\bar{z}}{\bar{w}+\bar{z}}{\right)`

=
`\frac{(w+z)(1+\bar{w}\bar{z})}{(1+wz)(\bar{w}+\bar{z})}=\frac{w+z+w\bar{w}\bar{z}+z\bar{z}\bar{w}}{\bar{w}+\bar{z}+\bar{w}wz+\bar{z}zw}=\frac{w+z+w+|w|^2\bar{z}+|z|^2\bar{w}}{\bar{w}+\bar{z}+|w|^2z+|z|^2w}=\frac{w+z+\bar{z}+\bar{w}}{\bar{w}+\bar{z}+z+w}=1`

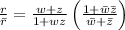
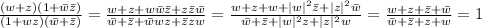
Thus
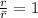`\frac{r}{\bar{r}}=1`. From this,
`r=\bar{r}`. A complex number is real if and only if it is equal to its conjugate, therefore r is real.