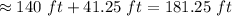Answer:
New dimensions of the floor is approximately 301.25 ft by 181.25 ft
Explanation:
The question is incomplete. The complete question should be:
Manufacture wants to enlarger it's floor area 1.5 times that of the current facility. The current facility is 260 ft by 140 ft. The manufacture wants to increase each dimension the same amount. Write the dimensions of the new floor.
Given:
Length of floor = 260 ft
Width of floor = 140 ft
The floor area is increased 1.5 times.
To find the new dimensions of the floor.
Solution:
Original area of the floor =
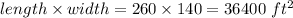
New area =
Let the length and width be increased by
 ft.
ft.
Thus, new length =
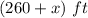
New width =
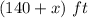
Area of the new floor can be given as:
⇒
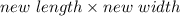
⇒
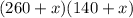
Multiplying using distribution.
⇒
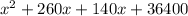
⇒
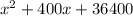
Thus we can equate this with new area to get the equation to find

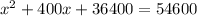
subtracting both sides by 54600.
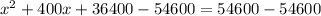

Using quadratic formula:
For a quadratic equation

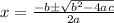
For the equation
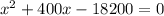
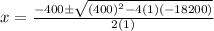
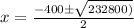

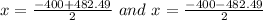
∴
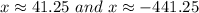
Since length is being increased, so we take
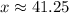
New dimensions are:
New length
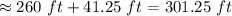
New width