Question

A certain radioactive isotope placed near a Geiger counter registers 120 counts per minute. If the half-life of the isotope is one day, what will the count rate be at the end of four days?

Answer 1

The count rate at the end of four days will be 7.5 counts per minute.

Step-by-step explanation:

First it is important to know that half-life is the time to a piece of radioactive material to decay 50%. So if we know we start with 120 counts per minute and we already know the half-life of the isotope is 1 hour we expect that past 1 hour the material decays 50% (it's halved) so we will count 60 counts per minute, now if we wait another hour 60 counts will decay in to 30 counts per minute and so on. That should be translate to a math equation as:

final counts = initial counts *
`((1)/(2))^(\#half\,life\,periods)`

After 4 days we have 4 half-life periods passed so:

final counts= 120 counts per minute *
`((1)/(2))^(4)`

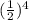
final material = 7.5 counts per minute