Answer:
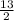 square units
square units
Explanation:
We are given that vertices of a right triangle are A(3,1) ,B(5,4) and C(6,-1).
We have to find the area of triangle.
We know that area of triangle=
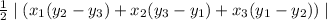
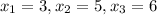
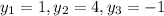
Substitute the values in the formula then we get
Area of right triangle =

Area of right triangle =
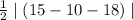
Area of right triangle =
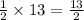 square units
square units