Question

Given f(x)=2x^2+3x-5 for what values of x is f(x) positive

Answer 1

The function f(x) is positive in the interval (-≠,-2.5) ∪ (1,∞)

Explanation:

we have

`f(x)=2x^(2)+3x-5`

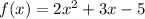
This is a vertical parabola open upward (the leading coefficient is positive)

The vertex is a minimum

The coordinates of the vertex is the point (h,k)

step 1

Find the vertex of the quadratic function

Factor the leading coefficient 2

`f(x)=2(x^(2)+(3)/(2)x)-5`

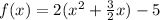
Complete the square

`f(x)=2(x^(2)+(3)/(2)x+(9)/(16))-5-(9)/(8)`

`f(x)=2(x^(2)+(3)/(2)x+(9)/(16))-(49)/(8)`

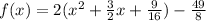
Rewrite as perfect squares

`f(x)=2(x+(3)/(4))^(2)-(49)/(8)`

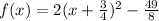
The vertex is the point (-\frac{3}{4},-\frac{49}{8})

step 2

Find the x-intercepts (values of x when the value of f(x) is equal to zero)

For f(x)=0

`2(x+(3)/(4))^(2)-(49)/(8)=0`

`2(x+(3)/(4))^(2)=(49)/(8)`

`(x+(3)/(4))^(2)=(49)/(16)`

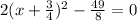
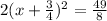
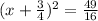
take the square root both sides

`x+(3)/(4)=\pm(7)/(4)`

`x=-(3)/(4)\pm(7)/(4)`

`x_1=-(3)/(4)+(7)/(4)=1`

`x_2=-(3)/(4)-(7)/(4)=-2.5`

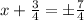
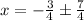
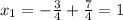
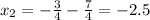
therefore

The function f(x) is negative in the interval (-2.5,1)

The function f(x) is positive in the interval (-≠,-2.5) ∪ (1,∞)

see the attached figure to better understand the problem