Question

Select all the points that are solutions to the system of linear inequalities that is listed below 10x + 4y < 12

8x - 3y > 20
. (3, -8) (2, 5) (-5, 1) (10, 3) (2, -10)

Answer 1

(3,-8)(2,-10)

Explanation:

Answer 2

(3,-8)

(2,-10)

Explanation:

we have

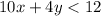`10x + 4y < 12` ----> inequality A

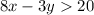`8x- 3y > 20` ----> inequality B

we know that

If a point is a solution of the system of inequalities, then the point must satisfy both inequalities (makes true both inequalities)

Verify all the points

Substitute the value of x and the value of y of each point in both inequalities

Case 1) point (3,-8)

For x=3, y=-8

inequality A

`10(3) + 4(-8) < 12`

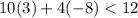
`-2 < 12` ----> is true

inequality B

`8(3)- 3(-8) > 20`

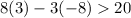
`48 > 20` ---> is true

therefore

The point is a solution of the system of linear inequalities

Case 2) point (2,5)

For x=2, y=5

inequality A

`10(2) + 4(5) < 12`

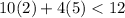
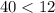`40 < 12` ----> is not true

therefore

The point is not a solution of the system of linear inequalities

Case 3) point (-5,1)

For x=-5, y=1

inequality A

`10(-5) + 4(1) < 12`

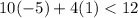
`-46 < 12` ----> is true

inequality B

`8(-5)- 3(1) > 20`

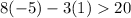
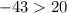`-43 > 20` ---> is not true

therefore

The point is not a solution of the system of linear inequalities

Case 4) point (10,3)

For x=10, y=3

inequality A

`10(10) + 4(3) < 12`

`112 < 12` ----> is not true

therefore

The point is not a solution of the system of linear inequalities

Case 5) point (2,-10)

For x=2, y=-10

inequality A

`10(2) + 4(-10) < 12`

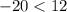`-20 < 12` ----> is true

inequality B

`8(2)- 3(-10) > 20`

`46 > 20` ---> is true

therefore

The point is a solution of the system of linear inequalities

see the attached figure to better understand the problem

If a ordered pair is a solution of the system , then the ordered pair must lie in the shaded area of the solution set