Answer:

Explanation:
A. Scale factor
When you dilate an object by a scale factor, you multiply its line lengths by the same number.
If EF/AB = 13/7, the scale factor is 13/7.
B. Length of EF
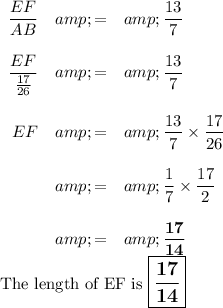
C. Area of EFGH
If the lengths in a shape are all multiplied by a scale factor, then the areas will be multiplied by the scale factor squared.
ABCD is dilated by a scale factor of 13/7, so its area is dilated by a scale factor of
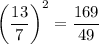
The area of its dilated image EFGH is
