Answer:
The Amount paid after 8 years is $72611.76
Explanation:
Given as :
The principal mortgage = p = $31,200
The rate of interest = r = 10.7% compounded quarterly
The time period of mortgage = t = 8 years
Let The Amount paid after 8 years = $A
Now, According to question
From Compounded Interest method
Amount = Principal ×
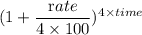
Or, A = p ×

Or, A = $31,200 ×
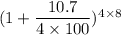
Or, A = $31,200 ×
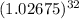
Or, A = $31,200 × 2.3273
∴ A = $72611.76
So, The Amount paid after 8 years = A = $72611.76
Hence, The Amount paid after 8 years is $72611.76 Answer