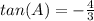Answer:
Part 1)
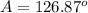
Part 2)
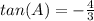
Explanation:
we have

The cos(A) is negative, that means that the angle A in the triangle ABC is an obtuse angle and the value of the sin(A) is positive
The angle A lie on the II Quadrant
step 1
Find the measure of angle A

using a calculator
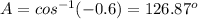
step 2
Find the sin(A)
we know that
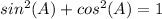
substitute the value of cos(A)
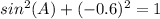
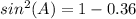
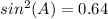
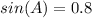
step 3
Find tan(A)
we know that
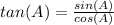
substitute the values
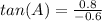
Simplify