Answer: 5.17 m
Step-by-step explanation:
The football reaches its maximum height when the vertical component of the velocity is zero:
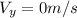
This is exactly at the point where the football stops and then begins to fall thanks to the acceleration due gravity.
So, we can use the following equation:
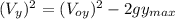
Where:
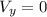 is the final velocity
is the final velocity
 is the vertical component of the initial velocity
is the vertical component of the initial velocity
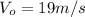 is the initial velocity
is the initial velocity
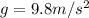 is the acceleration due gravity
is the acceleration due gravity
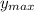 is the football's maximum height
is the football's maximum height
Isolating
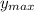 :
:
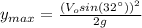
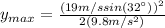
Finally:
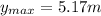 This is the football's maximum height
This is the football's maximum height