Question

What is the solution to the equation 5a^2 - 44 = 81

A. Plus minus 25
B. -5
C. Plus minus 5
D. Plus minus 125

Answer 1

Option c) is correct

ie, a=plus minus 5 or
`a=\pm 5`

Explanation:

Given equation is
`5a^2-44=81`

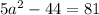
To find the solution of the given equation we have to solve the equation

`5a^2-44=81`

`5a^2=81+44`

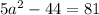
Now applying the algebraic sum to the terms on the right hand side of the equation

`5a^2=125`

`a^2=(125)/(5)`

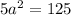
Now applying the division rule

`a^2=25`

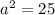
Therefore
`a=\pm 5`

Therefore option c) is correct

ie,
`a=\pm 5`.

Answer 2

Explanation:

5a^2 - 44 = 81

5a^2 = 81 + 44 {add 44 both the sides}

5a^2 = 125

a^2 = 125/5 {divide both sides by 5}

a^2 = 25

a^2 = 5*5

a = ±5