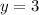Question

Solve the system:
5x-7y=-41
-3x-5y=-3

Answer 1

x = -4 , y = 3

Explanation:

5x - 7y = -41 ... (i)

-3x - 5y = -3 ... (ii)

Multiplying (i) by -3 and (ii) by 5 ;

-15x + 21y = 123 ... (i)

-15x - 25y = -15 ... (ii)

Subtracting (i) by (ii) ;

0 + 46y = 138

46y = 138

y = 138 ÷ 46 = 3

Returning to equation (ii) ;

-3x - 5(3) = -3

-3x = -3 + 15

-3x = 12

x = -4

Answer 2

Answer: The values of x and y in the given equations are
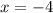`x=-4` and
`y=3`

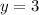
Step by step explanation:

Given system of equations are

`5x-7y=-41\hfill (1)`

`-3x-5y=-3\hfill (2)`

To find the values of x and y :

by using Elimination method

Now multiplying the equation (1) into 3 we get

`15x-21y=-123`

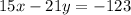
Now multiplying the equation (2) into 5 we get

`-15x-25y=-15`

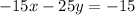
Adding the above two equations

`15x-21y=-123`

`-15x-25y=-15`

_________________

`-46y=-138`

_________________

`46y=138`

`y=(138)/(46)`

`y=3`

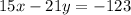
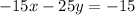
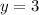
Therefore
`y=3`

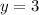
Substitute y value in equation (1)

`5x-7y=-41`

`5x-(7* 3)=-41`

`5x-21=-41`

`5x=21-41`

`5x=-20`

`x=-(20)/(5)`

`x=-4`

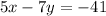
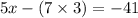
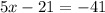
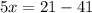
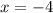
Therefore
`x=-4`

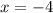
The values of x and y are
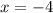`x=-4` and
`y=3`