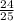Question

If cosA = 3/5 and A ∈ (630,720), find sin2A

Answer 1

-
`(24)/(25)`

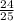
Explanation:

Given 630 < A > 720 then A is in the fourth quadrant where

cosA > 0 and sinA < 0

Given

cosA =
`(3)/(5)` =
`(adjacent)/(hypotenuse)`

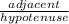
Then the triangle is a 3- 4 - 5 with opposite side 4, thus

sinA = -
`(opposite)/(hypotenuse)` = -
`(4)/(5)`

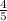
Using the trigonometric identity

sin2A = 2sinAcosA

= 2 × -
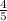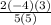`(4)/(5)` ×
`(3)/(5)`

=
`(2(-4)(3))/(5(5))` = -
`(24)/(25)`