Answer:

Explanation:
This question involves some basic knowledge of trigonometric function. The following formula only works for right angled triangles.
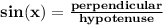
In ΔAKO,
m∠AKO = 90°
Therefore ΔAKO is a right angled triangle. If we take ∠A into consideration then base will be AK, perpendicular will be OK and hypotenuse will be AO.
AO = 15
OK = 5


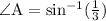
To calculate
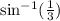 use scientific calculator
use scientific calculator
value of
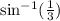 is approximately equal to 19.47°
is approximately equal to 19.47°
Therefore
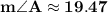
(NOTE : When a line passing through center of a circle, is drawn to a tangent at the point of tangency then the angle made between then is 90°
Therefore m∠AKO = 90°)