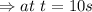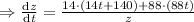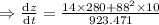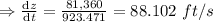Answer:
Explanation:
Given
Balloon velocity is 14 ft/s upwards
Distance between balloon and cyclist is 140 ft
Velocity of motor cycle is 88 ft/s
After 10 sec
motorcyclist traveled a distance of
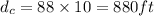
Distance traveled by balloon in 10 s
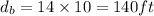
net height of balloon from ground =140+140=280 ft[/tex]
at
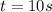
distance between cyclist and balloon is
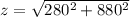
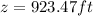
now suppose at any time t motorcyclist cover a distance of x m and balloon is at a height of h m
thus
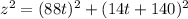
differentiating w.r.t time