Answer:
The numbers are -14,-13 and -12
Explanation:
The correct question is
Find three consecutive integers such that the sum of the first, twice the second and three times the third is -76. What is the number?
Let
x ----> the first consecutive integer
x+1 ---> the second consecutive integer
x+2 ---> the third consecutive integer
we have that
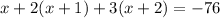
solve for x
apply distributive property
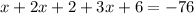
Combine like terms

subtract 8 both sides
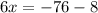
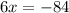
Divide by 6 both sides
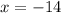
so
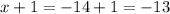
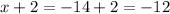
therefore
The numbers are -14,-13 and -12