Question

In ordered pair a solution
`y\geq x-5?`

Answer 1

The graph is plotted below.

One ordered pair as solution is (0, 0).

Explanation:

Given:

The inequality is given as:

`y\geq x-5`

In order to plot it, we first replace '≥' by '=' sign. This gives,

`y = x-5`

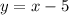
Now, we plot the above line. For that, we need the x and y intercepts.

At x-intercept, y = 0. So,

`0=x-5\\x=5`

The point is (5, 0)

At y-intercept, x = 0. So,

`y=0-5\\y=-5`

The point is (0, -5)

Now, plot these two points and draw a line passing through these two points. The graph is shown below.

Now, replace '=' by '≥' sign. Since, 'y' is greater than equal to 'x - 5'. So, the solution region is above the given including the values on the line.

Therefore, any ordered pair in the solution region is a solution of the given inequality. From the graph, one such ordered pair is (0, 0).

This can be verified from the inequality also.

`0\geq 0-5\\0\geq -5(True)`

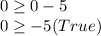
So, one ordered pair is (0, 0).