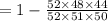Answer:
Explanation:
Given
There are 52 cards in total
there are total of 13 pairs of same cards with each pair containing 4 cards
Probability of getting a pair or three of kind card=1-Probability of all three cards being different
Probability of selecting all three different cards can be find out by selecting a card from first 13 pairs and remaining 2 cards from remaining 12 pairs i.e.
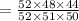
for first card there are 52 options after choosing first card one pair is destroyed as we have to select different card .
For second card we have to select from remaining 12 pairs i.e. 48 cards and so on for third card.
Required Probability is