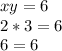Question

If 5x=y+75x=y+7, is (x−y)>0(x−y)>0? (1) xy=6xy=6 (2) xx and yy are consecutive integers with the same sign

Answer 1

No. If 5x=y+7 then xy=6 and (2) x and y are consecutive integers with the same sign. for xy=6

Explanation:

For the sake of clarity:

If 5x=y+7 then (x – y) > 0?

Alternatives:

(1) xy = 6

(2) x and y are consecutive integers with the same sign

1) Consider (x-y)>0 as true:

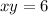`xy=6` Numbers like, 3*2, 6*1, etc..

`5x=y+7\Rightarrow (5x)/(5)=(y+7)/(5)\Rightarrow x=(y+7)/(5)\\Plugging\: in:\:\\(y+7)/(5)-y>0\Rightarrow (y+7-5y)/(5)>0\Rightarrow (-4y+7)/(5)>0\Rightarrow (-4y+7)/(5)*5>0*5\\-4y+7>0 *(-1)\Rightarrow 4y-7<0\:y>(7)/(4)\therefore y<1.75`

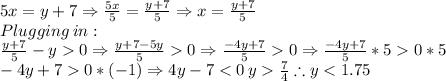
Since y in this hypothetical case is lesser then let's find x, let's plug in y 1 for a value lesser than 1.75:

Then xy≠6 and no and 8/5 (1.75) is a rational number. What makes false the second statement about consecutive integers.

So this is a Contradiction. (x-y) >0 is not true for 5x=x+7.

2) Consider:

x and y are consecutive integers with the same sign is true.

Algebraically speaking, two consecutive integers with the same sign can be written as:

`y=x+1`

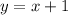
Plugging in the first equation (5x=y+7):

5x=x+1+7⇒4x=8 ⇒x =2

Since y=3 then x=2 because:

`3=x+1\\3-1=x+1-1\\2=x \Rightarrow x=2`

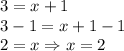
3) Testing it

`5x=y+7\\\\5(2)=(3)+7\\\\10=10\:True`

`xy=6\\2*3=6\\6=6`