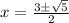Question

Use the quadratic formula to find the solutions to the equation.
7- 3x+1=0

Answer 1

B

Explanation:

quadratic formula:

x = [-b ± sqrt(b^2 - 4ac)] / 2a

1. find a, b, and c

a = 1

b = -3

c = 1

2. plug values into formula

x = [3 ± sqrt(9-4•1•1)] / 2

3. simplify

x = [3 ± sqrt(5)] / 2

This is B.

Answer 2

Option b) is correct

ie.,
`x=(3\pm √(5))/(2)`

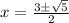
Explanation:

Given quadratic equation is
`x^(2)-3x+1=0`

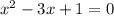
To find the solutions of given equation:

Solution of quadratic equation
`ax^(2)+bx+c=0`

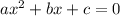
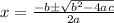`x=\frac{-b\pm \sqrt{b^(2)-4ac}}{2a}` where a,b, are coefficients of
`x^(2)` and x respectively and c is constant.

From the given quadratic equation a=1, b=-3 and c=1

`x=\frac{-(-3)\pm \sqrt{(-3)^(2)-4(1)(1)}}{2(1)}`

`x=(3\pm √(9-4))/(2)`

`x=(3\pm √(5))/(2)`

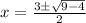
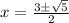
Therefore Option b) is correct

ie.,
`x=(3\pm √(5))/(2)`