Question

If $-5\leq a \leq -1$ and $1 \leq b \leq 3$, what is the least possible value of $\displaystyle\left(\frac{1}{a} \frac{1}{b}\right)\left(\frac{1}{b}-\frac{1}{a}\right) $

Answer 1

The least possible value is -2, obtained for a = -1 and b = 1.

Explanation:

We want the minimum of
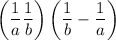`\displaystyle\left((1)/(a) (1)/(b)\right)\left((1)/(b)-(1)/(a)\right)` , where

• 
  `-5 \leq a \leq -1`
• 
  `1 \leq b \leq 3`

First, lets simplify the expression given, we use common denominator on the second part, using ab as the common denominator. We obtain

`(1)/(b) - (1)/(a) = (a-b)/(ab)`

As a result

`\displaystyle\left((1)/(a) (1)/(b)\right)\left((1)/(b)-(1)/(a)\right) = (a-b)/((ab)^2)`

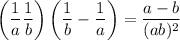
we need the minimum of the function

`f(a,b) = (a-b)/((ab)^2)`

with the restrictions
`-5 \leq a \ -1, 1 \leq b \leq 3`

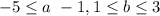
First, we calculate the gradient of f and find where it takes the zero value.

`\\abla{f} = (f_a,f_b)`

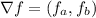
with

`f_a = ((ab)^2 - (a-b) 2ab^2)/((ab)^2) = -1 + 2 (b)/(a)`

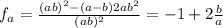
Since it has the reversed sign, we get

`f_b = - (-1 + 2 (a)/(b)) =1 - 2 (a)/(b)`

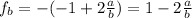
In order for
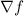`\\abla{f}` to be zero, we need both
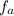`f_a` and
`f_b` to be zero, observe that

`f_b = 0 \, \rightarrow 1 -2(a)/(b) = 0 \, \rightarrow 1 = 2 (a)/(b) \, \rightarrow b = 2a`

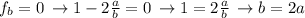
Which is impossible with the given restrictions. Hence, the minimum is realized in the border.

If we fix a value a₀ for a, with a₀ between -5 and -1 the function g(b) = f(a₀,b) wont have a minimum for b in [1,3] because the partial derivate of f over b didnt reach the value 0 in the restrictions given. On the other hand. by making a similar computation that before, we can obtain that the partial derivate of f over the variable a doesnt reach the value 0 either. This means that f doesnt reach the minimum on the sides. As a consecuence, it reach a minimum on the corners.

The 4 possible corner values are (-5,1), (-5,3), (-1,1) and (-1,3)

`f(-5,1) = (-6)/(25) =  -0.24`

`f(-5,3) = (-8)/(225) = -0.0355`

`f(-1,1) = (-2)/(1) = -2`

`f(-1,3) = (-4)/(9) = -0.444`

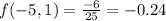
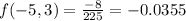
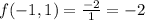
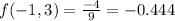
Clearly the least possible value between the four corners is -2.