Answer:
In the quotient
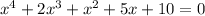 , the coefficient of x is 5.
, the coefficient of x is 5.
Explanation:
Given rational expression is
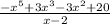
Nicolette used synthetic division to solve the given rational expression.
Nicolette's work is given below:
Given that x-2 is a zero of given rational expression
By using synthetic division for the given rational expression.
2_| -1 0 3 -3 0 20
0 -2 -4 -2 -10 -20
____________________________
-1 -2 -1 -5 -10 | 0
Therefore the simplified expression can be written as below
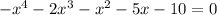
Taking negative sign outside.
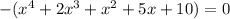
Multiply negative sign with "0" we get "0"
Therefore
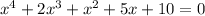
In the quotient
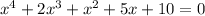 , the coefficient of x is 5.
, the coefficient of x is 5.