Answer:
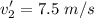
Step-by-step explanation:
Conservation Of Linear Momentum
The total momentum of both balls won't change regardless of their interaction while no external forces are acting on the system. We'll use the following variables m1,m2,v1,v2,v1',v2' for the mass of the white ball, the mass of the red ball, their velocities before the collision, and their velocities after the collision, respectively .
The provided data is as follows:
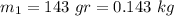
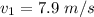
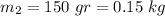
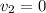
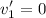
To preserve the total linear momentum, the following equation must stand
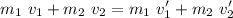
Solving for
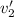
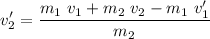
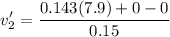
The final speed of the red ball is
