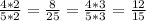Question

15. Mr. Clements painted his barn for hours in the morning. He painted

the barn for 5 hours in the afternoon. For 154-15c, select True or False

for each statement.

15a. A common denominator of

the mixed numbers is 20.

O True

False

O True

O False

15b. The amount of time spent

painting in the morning can be

rewritten as 3 hours.

False

15c. Mr. Clements spent 2 hours O True

longer painting in the afternoon

than the morning

16. Tom exercised hour on Monday and hour on Tuesday.

Answer 1

15a) True 15b) False 15c) True

16)
`(4*2)/(5*2)=(8)/(25)=(4*3)/(5*3)=(12)/(15)`

`(5*2)/(6*2)=(10)/(12)=(5*3)/(6*3)=(15)/(18)`

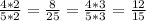
Explanation:

*Rewriting the question with Retrieved missing information:

15. Mr. Clements painted his barn for hours in the morning. He painted his barn
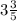`3(3)/(5)` hours in the morning. He painted the barn for
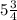`5(3)/(4)` hours in the afternoon.Select True or False for each statement.

15a. A common denominator of the mixed numbers is 20.

15b. The amount of time spent painting in the morning can be rewritten as
`3(15)/(20)`

15c. Mr. Clements spent
`2(3)/(20)`

hours longer painting in the afternoon than the morning.

16. Tom exercised:

`(4)/(5)`

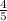
hour on Monday and

`(5)/(6)`

hour on Tuesday. Part A. Complete the calculations below to write equivalent fractions with a common denominator.

15a) True

Turning the Mixed Numbers into Improper ones:

He painted the his barn:

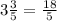`3(3)/(5)=(18)/(5)` Keep the denominator, and for the numerator multiply the denominator 5 by 3 and add 3 = 18. (In the morning).

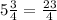`5(3)/(4)=(23)/(4)` (In the afternoon)

The Prime factorization
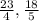`(23)/(4),(18)/(5)` help us to obtain the common denomination, i.e. 20

15b) False

This is a matter of simplification. In this mixed number, the fraction can be simplified this way:

`3(15)/(20)=3(15:5)/(20:5)\Rightarrow 3(3)/(4)`

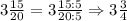
15c) True

Subtracting the working hours, knowing the common denominator by prime factorization is 20.

For Mixed Numbers:

`5(3)/(4)-3(3)/(5)=5(15)/(20)-3(12)/(20)=2(3)/(20)`

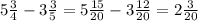
For the same mixed numbers turned into improper fractions:

`(23)/(4)-(18)/(5)=(115-72)/(20)=(43)/(20)`

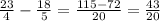
Notice that:

`2(3)/(20)==(43)/(20)=2.15`

16A

Equivalent fractions are fractions multiplied by a same constant, on the numerator and denominator:

`(4*2)/(5*2)=(8)/(25)=(4*3)/(5*3)=(12)/(15)`

`(5*2)/(6*2)=(10)/(12)=(5*3)/(6*3)=(15)/(18)`