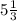 liters is the amount to be drained out and replaced
liters is the amount to be drained out and replaced
Solution:
40 % antifreeze solution in 16 liter radiator
Let "x" be the amount drained from radiation and replaced with pure antifreeze
To obtain a 60 % antifreeze solution
The original solution is 16 liter, 40% of which is antifreeze
You want the solution to be 60% antifreeze:
60 % x 16 =
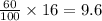
You will remove x liters of the 40% solution and replace it with x liters pure (100%) antifreeze.
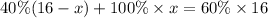
Let us solve expression for "x"
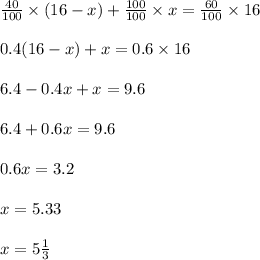
Thus
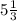 liters is the amount to be drained out and replaced
liters is the amount to be drained out and replaced