Answer:
4.192 N
Step-by-step explanation:
Step 1: Identify the given parameters
Velocity of airflow = 45m/s
air temperature = 20⁰
plate length and width = 1m and 0.5m respectively.
Step 2: calculate drag force due to shear stress,
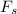

Note: The density and kinematic viscosity of air at 20⁰ at 1 atm, is 1.2 kg/m³ and 1.5 X 10⁻⁵ N.s/m²
⇒The Reynolds number (
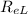 ) based on the length of the plate is
) based on the length of the plate is
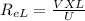
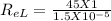
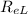 = 3 X10⁶ (flow is turbulent, Re ≥ 500,000)
= 3 X10⁶ (flow is turbulent, Re ≥ 500,000)
⇒The average shear stress coefficient (
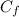 ) on the "tripped" side of the plate is
) on the "tripped" side of the plate is
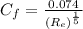
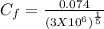
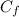 = 0.0038
= 0.0038
⇒The average shear stress coefficient (
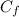 ) on the "untripped" side of the plate is
) on the "untripped" side of the plate is
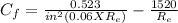
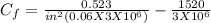
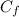 = 0.0031
= 0.0031
The total drag force =
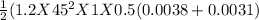
The total drag force is 4.192 N