Question

A single-threaded power screw is 25 mm in diameter with a pitch of 5 mm. A vertical load on the screw reaches a maximum of 5 kN. The coefficients of friction are 0.06 for the collar and 0.09 for the threads. The frictional diameter of the collar is 45 mm. Find the overall efficiency and the torque to ""raise"" and ""lower"" the load.

Answer 1

0.243

Step-by-step explanation:

Step 1: Identify the given parameters

Force (f) = 5kN, length of pitch (L) = 5mm, diameter (d) = 25mm,

collar coefficient of friction = 0.06 and thread coefficient of friction = 0.09

Frictional diameter =45mm

Step 2: calculate the torque required to raise the load

`T_(R) = (5(25))/(2) [(5+\pi(0.09)(25))/(\pi(25)-0.09(5))]+(5(0.06)(45))/(2)`

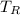`T_(R)` = (9.66 + 6.75)N.m

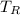`T_(R)` = 16.41 N.m

Step 3: calculate the torque required to lower the load

`T_(L) = (5(25))/(2) [(\pi(0.09)(25) -5)/(\pi(25)+0.09(5))]+(5(0.06)(45))/(2)`

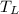`T_(L)` = (1.64 + 6.75)N.m

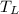`T_(L)` = 8.39 N.m

Since the torque required to lower the thread is positive, the thread is self-locking.

The overall efficiency =
`(F(L))/(2\pi(T_(R)))`

=
`(5(5))/(2\pi(16.41))}`

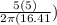
= 0.243