Question

A sporting goods stores sells footballs, basketballs, and volleyballs. A football costs $35, a basketball costs

s , and a volleyball costs $15. On a given day, the store sold 5 times as many footballs as volleyballs. They

brought in a total of $3750 that day, and the money made from basketballs alone was 4 times the money

made from volleyballs alone. How many footballs, basketballs, and volleyballs were sold? Just set up the

problem

Answer 1

The number of footballs, basketballs and volleyballs were sold are 75, 36 and 15 respectively.

Explanation:

Consider the provided information.

A football costs $35, a basketball costs $25 and a volleyball costs $15.

Let F represents the football, B represents the basketball and V represents the volleyball.

On a given day, the store sold 5 times as many footballs as volleyballs.

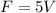`F=5V`......(1)

They brought in a total of $3750 that day,

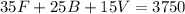`35F+25B+15V=3750`......(2)

The money made from basketballs alone was 4 times the money.

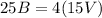`25B=4(15V)`......(3)

By equation 1, 2 and 3.

`35(5V)+4(15V)+15V=3750`

`250V=3750`

`V=15`

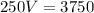
Substitute the value of V in equation 1 and 3.

`F=5(15)=75`

`25B=4(15* 15)\\B=36`

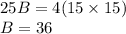
Hence, the number of footballs, basketballs and volleyballs were sold are 75, 36 and 15 respectively.