Question

After an exceptionally rainy spring, there is no longer a barrier between the populations, and some individuals migrate inland from the coast. Consequently, 20 % of individuals in the new inland population are originally from the coastal population. Calculate the spotted allele frequency for the inland population after migration, q combined . Round the answer to the nearest hundredth.

Answer 1

After inland population after migration allele frequency is 0.62 or 62%

Step-by-step explanation:

Given,

Coastal striped phenotype freq. = 0.22

ss = 0.22

`q_(coastal) * q_(coastal) = 0.22`

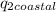`q_(2 coastal)`= 0.22

Similarly, inland striped phenotype freq. = 0.43

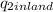`q_(2inland)` = 0.43

`q_(coastal) = \sqrt{q_(2coastal)}`

=
`√(0.22)`

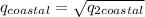
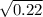
= 0.4690

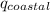`q_(coastal)`= 0.47 i.e. 47%

`q_(inland) = √(0.43)`

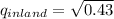
= 0.655

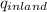`q_(inland)` = 0.66 i.e. 66%

the migration range (m) is given as 20%

m= 0.2

allele freq. after migration = pre migration + ∆q

here,

∆q = change in the allele frequency

or

migration of allele freq. from coastal to inland

=
`m(q_(coastal) - q_(inland))`

= 0.2 (0.47 – 0.66)

=
`0.2 * (- 0.191)`

= - 0.0382

∆q = -0.04 i.e. 4%