Answer:
The last is the correct option
"all real values except x not-equals 7 and the x for which f (x) not-equals negative 3"
Explanation:
Domain and Range of Functions
Given the function f(x), the domain of f is the set of all the values that x can take such f(x) exists. The range of f is the set of all the values that f takes.
We have a problem where we have to find the domain of a composite function. Let's recall that being f and g real functions, then
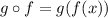
is the composite function of f and g.
We know the domain of f is the set of all real values except 7, and the domain of g is the set of all real values except –3.
Since f is the innermost function, the domain of the composite function is directly restricted by the domain of f. So, x cannot be 7.
Now, g takes f as its independent variable, and we know the domain of g excludes -3. It can be found that f(x) cannot be -3 because it will cause g not to exist.
Thus, the domain of
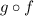 is
is
All real numbers except x=7 and those where f(x)=-3
The last is the correct option