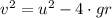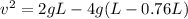Question

A peg is located a distance h directly below the point of attachment of the cord. If h = 0.760 L, what will be the speed of the ball when it reaches the top of its circular path about the peg?

Answer 1

Step-by-step explanation:

Given

Pivot is at h=0.76 L

Where L is the length of String

Conserving Energy at A and B

`mgL=(1)/(2)mu^2`

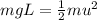
where u=velocity at bottom

`u=√(2gL)`

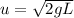
After coming at bottom the ball completes the circle with radius r=L-0.76 L

Suppose v is the velocity at the top

Conserving Energy at B and C

`(1)/(2)mu^2=mg(2r)+(1)/(2)mv^2`

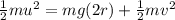
Eliminating m

`u^2=4r+v^2`

`v^2=u^2-4\cdot gr`

`v^2=2gL-4g(L-0.76L)`

`v^2=1.04gL`

`v=√(1.04gL)`