Question

A chemist currently has a solution of 30% hydrochloric acid and a solution of 15% hydrochloric acid. He mixes the two solutions together to create 24 oz of a 25% hydrochloric acid solution. How many ounces of the 30% hydrochloric acid solution did he use in order to obtain the 25% solution

Answer 1

16 ounces of the 30% hydrochloric acid solution is used in order to obtain the 25% solution.

Explanation:

Let amount of 30% ounces be 'x' and that of 15% ounces by 'y'.

Given:

Total amount on mixing both the solution = 24 oz

∴
`x+y=24\\x=24-y------------ 1`

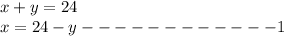
Also, the total acid content in the resulting 25% solution is equal to the sum of the acid contents in 30% and 15% solutions.

∴
`0.30x+0.15y=0.25(24)\\0.30x+0.15y=6-------2`

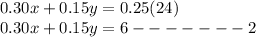
Now, plug in 'x' from equation (1) into equation (2). This gives,

`0.30(24-y)+0.15y=6`

`7.2-0.3y+0.15y=6`

`-0.3y-0.15y=6-7.2`

`-0.15y=-1.2`

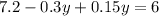
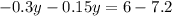
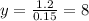`y=(1.2)/(0.15)=8` ounces

Therefore,
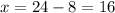`x=24-8=16` ounces

Hence, 16 ounces of the 30% hydrochloric acid solution is used in order to obtain the 25% solution