Explanation:
Refer to the given attachment for your better understanding .
As Per Provided Information
- AB = x
- BC = 4
- Angle formed on A = 60°
We have been asked to determine the value of x .
Using Trigonometric Ratio .
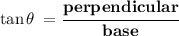
Substituting the value and let's solve it for x
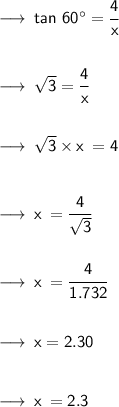
Therefore,
- Required value of x is 2.3
So your answer is (d) 2.3