Answer:
The area of the region inside the circumcircle of the triangle but outside the triangle is
![A=(27)/(4)[\pi-3√(3)]\ units^2](https://img.qammunity.org/2021/formulas/mathematics/middle-school/mgwcf0s4ws7750qrsb71mhdxuhm1ridhb9.png)
Explanation:
see the attached figure to better understand the problem
step 1
Find the area of triangle
we have an equilateral triangle
Applying the law of sines
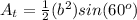
where b is the length side of the equilateral triangle
we have
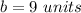
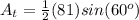
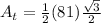

step 2
Find the area of circle
The area of the circle is equal to
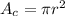
The formula to calculate the radius of the circumcircle of the triangle equilateral is equal to
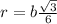
where b is the length side of the equilateral triangle
we have
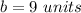
substitute


Find the area
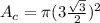
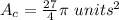
step 3
Find the area of the shaded region
we know that
The area of the region inside the circumcircle of the triangle but outside the triangle is equal to the area pf the circle minus the area of triangle
so
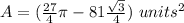
Simplify
![A=(27)/(4)[\pi-3√(3)]\ units^2](https://img.qammunity.org/2021/formulas/mathematics/middle-school/mgwcf0s4ws7750qrsb71mhdxuhm1ridhb9.png)