Question

What is the lateral area of a square pyramid with side length 11.2 and slant height 20

Answer 1

`448 cm^(2)`

Explanation:

s = 11.2; l = 20

L.A. =
`4 ((1)/(2) sl)`

L.A.=
`(1)/(2)(4 * 11.2)20` Multiply 4 * 11.2 to get perimeter 44.8

L.A. =
`(1)/(2) pl`

L.A. =
`(1)/(2) (44.8)20\\` Simplify 44.8 * 20

L.A. =
`(1)/(2)(896)\\` Divide 896 by 2

L.A. =
`448cm^(2)`

Answer 2

The lateral surface area of square pyramid is 448 square units.

Explanation:

We are given the following in the question:

Side length of square pyramid = 11.2 units

Slant height of square pyramid = 20 units

Lateral area of a square pyramid =

`L = (1)/(2)Ph`

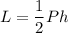
where P is the perimeter of square base and h is the slant height.

Perimeter of square base =

`P = 4* \text{Base edge}\\= 4* 11.2 = 44.8\text{ units}`

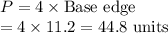
Putting the values, we get:

`L = (1)/(2)* 44.8* 20 = 448\text{ square units}`

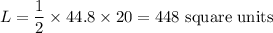
Thus, the lateral surface area of square pyramid is 448 square units.