Question

I NEED HELP WITH THESE QUESTIONS THANKYOUU

Answer 1

1. s = 19

2. 17

3. R

4. Angle bisector

5. 53°

Explanation:

1. If VY is a midsegment of triangle WXZ, then by midsegment theorem,

`VY=(1)/(2)WX.`

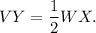
Since
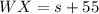`WX=s+55` and
`VY=s+18,` then

`s+18=(1)/(2)(s+55)\\ \\2(s+18)=s+55\\ \\2s+36=s+55\\ \\2s-s=55-36\\ \\s=19`

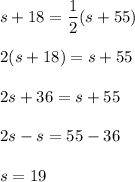
2. A triangle has two sodes of length 18 and 2 units.

Let c units be the length of the third side. Then

`18+c>2\\ \\2+c>18\\ \\2+18>c`

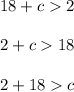
So,

`c>-16\\ \\c>16\\ \\c<20`

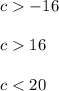
Hence,
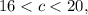`16<c<20,` and the smallest possible whole-number length for the third side is 17 units.

3. In triangle QSR, the smallest angle is opposite to the smallest side. The smallest side is the side with length of 49 m, then the smallest angle is angle R.

4. Given ∠VYW≅∠WYX.

Definition of angle bisector: An angle bisector in a triangle is a line segment that bisects one of the vertex angles of a triangle.

Thus, YW is angle Y bisector.

5. Consider two right triangles ACD and ABC. In these triangles,

• AC ≅ AC - reflexive property;
• AB ≅ AD - given.

Then, by HL postulate, triangles ACD and ABC are congruent. Congruent triangles have congruent corresponding sides, then

∠ACD ≅ ∠ACB

and

m∠ACB = m∠ACD = 53°