Answer:
![\sqrt[7]{x^(4)}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/ofv880i1706l8ctabkel50c8hewn4b2fkr.png)
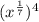
![(\sqrt[7]{x})^(4)](https://img.qammunity.org/2021/formulas/mathematics/middle-school/nilz49vl4yi8u8zjz7di3om5dk7c7j9ru5.png)
Explanation:
we have
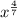
Remember the properties
![\sqrt[n]{a^(m)}=a^{(m)/(n)}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/7z57pg5r1vh1qpdj646hj8q6fudepod0rf.png)
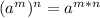
so
Verify each case
Part 1) we have
![\sqrt[4]{x^(7)}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/8zj4pxr0l6zd6onbus5s8cz1gkuvial9ce.png)
we know that
![\sqrt[4]{x^(7)}=x^{(7)/(4)}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/11ngo1cr5mdqokro39yuxyzkvhn5pn8zto.png)
Compare with the given expression
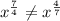
Part 2) we have
![\sqrt[7]{x^(4)}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/ofv880i1706l8ctabkel50c8hewn4b2fkr.png)
we know that
![\sqrt[7]{x^(4)}=x^{(4)/(7)}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/5epzqo0ffratcwip65sy18535uu7aoookd.png)
Compare with the given expression
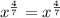
therefore
Is equivalent to the given expression
Part 3) we have
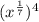
we know that

Compare with the given expression
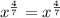
therefore
Is equivalent to the given expression
Part 4) we have
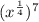
we know that
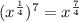
Compare with the given expression
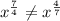
Part 5) we have
![(\sqrt[4]{x})^(7)](https://img.qammunity.org/2021/formulas/mathematics/middle-school/6wm3x43z2gyn2ezwcp1xm5uegtkqbr3u8g.png)
we know that
![(\sqrt[4]{x})^(7)=(x^{(1)/(4)})^(7)=x^{(7)/(4)}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/opm2lamdr1a0spo1cq1zgf4p0ft1qr9wlz.png)
Compare with the given expression
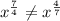
Part 6) we have
![(\sqrt[7]{x})^(4)](https://img.qammunity.org/2021/formulas/mathematics/middle-school/nilz49vl4yi8u8zjz7di3om5dk7c7j9ru5.png)
we know that
![(\sqrt[7]{x})^(4)=(x^{(1)/(7)})^(4)=x^{(4)/(7)}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/walr5sxi7ngz84z88bbz78jso1k2zw66xv.png)
Compare with the given expression
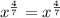
therefore
Is equivalent to the given expression