Question

The producer of a certain bottling equipment claims that the variance of all its filled bottles is .027 or less. A sample of 30 bottles showed a standard deviation of .2. The p-value for the test is _____.

a. .025
b. between .025 and .05
c. .05
d. between .05 and .01

Answer 1

`p_v = P(\chi^2_(29)>42.963)=1-0.954=0.0459`

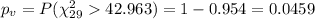
b. between .025 and .05

Explanation:

Previous concepts and notation

The chi-square test is used to check if the standard deviation of a population is equal to a specified value. We can conduct the test "two-sided test or a one-sided test".

`\bar X` represent the sample mean

n = 30 sample size

s= 0.2 represent the sample deviation

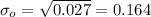`\sigma_o =√(0.027)=0.164` the value that we want to test

`p_v` represent the p value for the test

t represent the statistic

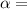`\alpha=` significance level

State the null and alternative hypothesis

On this case we want to check if the population standard deviation is less than 0.027, so the system of hypothesis are:

H0:
`\sigma \leq 0.027`

H1:
`\sigma >0.027`

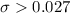
In order to check the hypothesis we need to calculate the statistic given by the following formula:

`t=(n-1) [(s)/(\sigma_o)]^2`

This statistic have a Chi Square distribution distribution with n-1 degrees of freedom.

What is the value of your test statistic?

Now we have everything to replace into the formula for the statistic and we got:

`t=(30-1) [(0.2)/(0.164)]^2 =42.963`

What is the approximate p-value of the test?

The degrees of freedom are given by:

`df=n-1= 30-1=29`

For this case since we have a right tailed test the p value is given by:

`p_v = P(\chi^2_(29)>42.963)=1-0.954=0.0459`

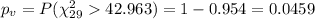
And the best option would be:

b. between .025 and .05