Answer:
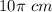 or
or

Explanation:
step 1
Find the circumference of the circle
The circumference of a circle is equal to
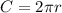
we have

substitute
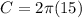
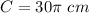
step 2
Remember that the circumference of the circle subtends a central angle of 360 degrees
so
using proportion
Find the length of an arc by a central angle of 120 degrees
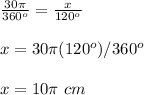
The exact value of the length of the arc is
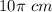
assume

 ---> approximate value
---> approximate value