Answer:
409.87803 m/s
Step-by-step explanation:
v = Velocity of bullet
L = Latent heat of fusion = 6 cal/g
c = Specific heat of lead = 0.03 cal/g°C
 = Change in temperature = (327-27)
= Change in temperature = (327-27)
m = Mass of bullet
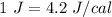
The heat will be given by the kinetic energy of the bullet
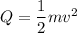
According to the question
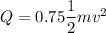
This heat will balance the heat going into the obstacle
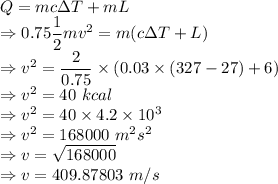
The speed of the bullet is 409.87803 m/s