Question

$13,957 is invested, part at 7% and the rest at 6%. If the interest earned from the amount invested at 7% exceeds the interest earned from the amount invested at 6% by $833.73, how much is invested at each rate?

Answer 1

The Amount invested at 7% interest is $12,855

The Amount invested at 6% interest = $1,102

Explanation:

Given as :

The Total money invested = $13,957

Let The money invested at 7% =
`p_1` = $A

And The money invested at 6% =
`p_2` = $13957 - $A

Let The interest earn at 7% =
`I_1`

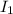
And The interest earn at 6% =
`I_2`

`I_1` -
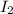
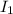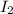`I_2` = $833.73

Let The time period = 1 year

Now, From Simple Interest method

Simple Interest =
`(\textrm principal* \textrm rate* \textrm time)/(100)`

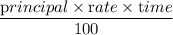
Or,
`I_1` =
`(\textrm p_1* \textrm 7* \textrm 1)/(100)`

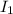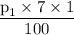
Or,
`I_1` =
`(\textrm A* \textrm 7* \textrm 1)/(100)`

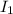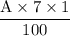
And

`I_2` =
`(\textrm p_2* \textrm 6* \textrm 1)/(100)`

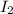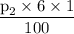
Or,
`I_2` =
`(\textrm (13,957 - A)* \textrm 6* \textrm 1)/(100)`

∵
`I_1` -
`I_2` = $833.73

So,
`(\textrm A* \textrm 7* \textrm 1)/(100)` -
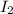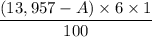
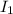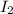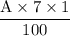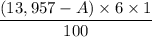`(\textrm (13,957 - A)* \textrm 6* \textrm 1)/(100)` = $833.73

Or, 7 A - 6 (13,957 - A) = $833.73 × 100

Or, 7 A - $83,742 + 6 A = $83373

Or, 13 A = $83373 + $83742

Or, 13 A = $167,115

∴ A =
`(167115)/(13)`

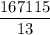
i.e A = $12,855

So, The Amount invested at 7% interest = A = $12,855

And The Amount invested at 6% interest = ($13,957 - A) = $13,957 - $12,855

I.e The Amount invested at 6% interest = $1,102

Hence,The Amount invested at 7% interest is $12,855

And The Amount invested at 6% interest = $1,102 . Answer