Question

If a_1=6a1​=6 and a_n=a_{n-1}+3an​=an−1​+3 then find the value of a_4a4​

Answer 1

The value of
`a_(4)=15`

Explanation:

Given that
`a_(1)=6` and
`a_(n)=a_(n-1)+3`

Given sequence is of the form arithmetic sequence

For arithmetic sequence the sequence is
`a_(1),a_(2),a_(3),...`

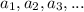
The nth term is of the form
`a_(n)=a_(n-1)+d`

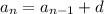
Here
`a_(1)=6` and
`a_(n)=a_(n-1)+3`

from this the common differnce is 3.

Therefore d=3

To find
`a_(2)`,
`a_(3)` ,
`a_(4)`

`a_(n)=a_(n-1)+d`

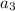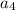
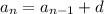
put n=2 and d=3 we get

`a_(2)=a_(2-1)+3`

`a_(2)=a_(1)+3`

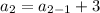
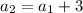
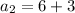`a_(2)=6+3` (here
`a_(1)=6` )

Therefore
`a_(2)=9`

`a_(n)=a_(n-1)+d`

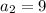
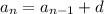
put n=3 and d=3 we get

`a_(3)=a_(3-1)+3`

`a_(3)=a_(2)+3`

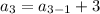
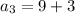`a_(3)=9+3` (here
`a_(2)=9` )

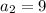Therefore
`a_(3)=12`

`a_(n)=a_(n-1)+d`

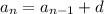
put n=4 and d=3 we get

`a_(4)=a_(4-1)+3`

`a_(4)=a_(3)+3`

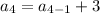
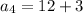`a_(4)=12+3` (here
`a_(3)=12` )

Therefore
`a_(4)=15`

Therefore the sequence is 6,9,12,15,...

Therefore the value of
`a_(4)=15`