Question

The surface area of the rectangular prism can be found using the expression 6x exponent 3 + 4x exponent 2 + 12x. if the value of x is 3, what is the surface area, in square centimeters of the prism?

Answer 1

234 cm²

Explanation:

Given expression to represent the surface area of the rectangular prism is
`6x^(3) +4x^(2) +12x`

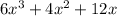
The given value of x is 3.

Now, finding value of expression by sustituting value of "x" as given.

Surface area of the rectangular prism =
`6x^(3) +4x^(2) +12x`

Surface area of the rectangular prism=
`6* 3^(3) +4* 3^(2) +12* 3`

∴ Surface area of the rectangular prism=
`6* 27+ 4* 9+ 12* 3`

Surface area of the rectangular prism=
`162+36+36= 234\ cm^(2)`

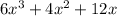
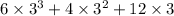
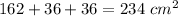
∴ Surface area of the rectangular prism= 234 cm²